 Electron. J. Probab. 23 (2018), no. 103, 16 pp.
Electron. J. Probab. 23 (2018), no. 103, 16 pp.
The natural parametrization of the SLE$_\kappa$ curve is the natural volume measure supported on this curve of Hausdorff dimension $1+ \kappa/8$ (for $\kappa\leq 8$). It can be constructed directly by counting the number of ball of small diameter needed to cover certain aprts of the curve. In this paper, we provide another construction of the natural parametrization of SLE$_\kappa$ for $\kappa<4$. We construct it as the expectation of the quantum time, which is a volume measure carried by SLE in an environment carrying a random metric built from a Gaussian free field. This point of view moreover provides another proof that natural parametrization is characterized by its Markovian covariance property.
We formulate a classification conjecture for conformally invariant families of measures on simple loops that builds on a conjecture of Kontsevich and Suhov. The main example in this class of objects was constructed by Werner as boundaries of Brownian loops. We present partial results towards the algebraic step of this classification.
Solving this conjecture would provide another argument explaining why planar statistical mechanics models with conformally invariant scaling limits naturally occur in a one-parameter family, together with the dynamical characterization of SLE via Schramm's central limit argument, and with the conformal field theory point of view and its central charge parameter.
Solving this conjecture would provide another argument explaining why planar statistical mechanics models with conformally invariant scaling limits naturally occur in a one-parameter family, together with the dynamical characterization of SLE via Schramm's central limit argument, and with the conformal field theory point of view and its central charge parameter.
 To appear in Annals of Probability
To appear in Annals of Probability
We consider the set of interfaces between $+$ and $-$ spins arising for the critical planar Ising model on a domain with $+$ boundary conditions, and show that it converges towards the collection of loops CLE$_3$.
Our proof relies on the study of a coupling between the Ising model and its random cluster (FK) representation, and of the interactions between FK and Ising interfaces. The main idea is to construct an exploration process starting from the boundary of the domain, to discover the Ising loops and to establish its convergence to a conformally invariant limit. The challenge is that Ising loops do not touch the boundary; we use the fact that FK loops touch the boundary (and hence can be explored from the boundary) and that Ising loops in turn touch FK loops, to construct a recursive exploration process that visits all the macroscopic loops.
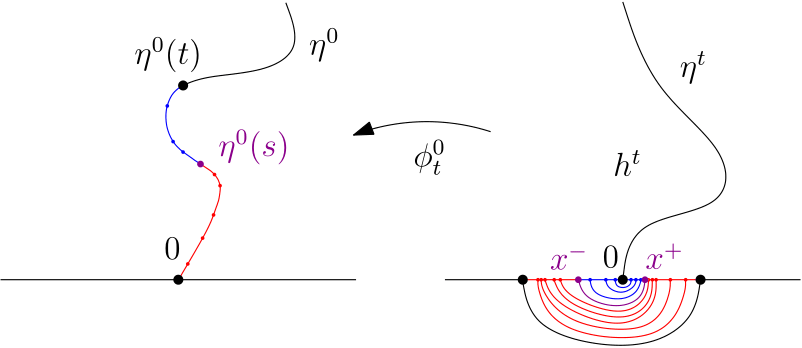 The Gaussian free field (GFF) conjecturally describes the scaling limit of discrete geometry. Typically, one studies the uniform measure on a certain class of large planar graphs equipped with their graph metric.
It is possible to decorate these graphs by drawing statistical mechanics models on them,
and thus reweighing the measure on metric spaces by the partition functions of a statistical mechanics model. The scaling limit of these decorated graphs can be conjecturally
described as a random metric space determined by a GFF, that moreover carries interfaces
of a model of statistical mechanics. In this way, SLE curves naturally appear in a coupling
with a GFF. In certain discrete settings, one can find a measure-preserving operation that
consists in cutting and opening up the graph alongside an interface of the statistical model
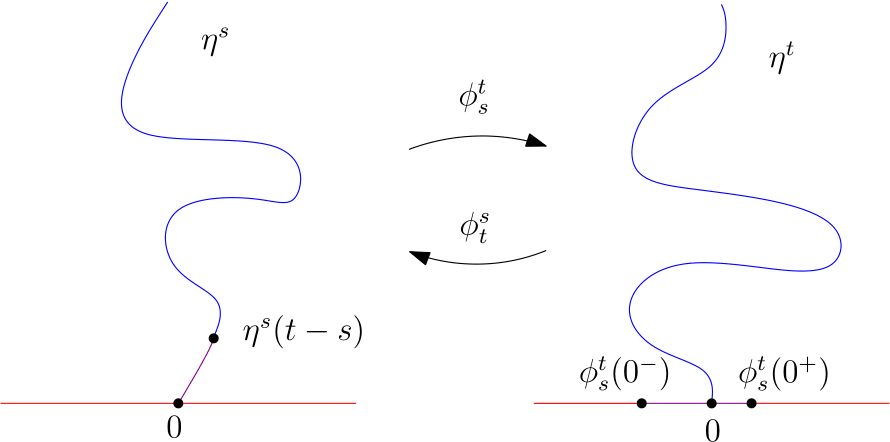
drawn on it. This discrete cutting process conjecturally converges to a continuous stationary process that we now describe. Given a Neumann free field in the upper half plane $\mathbb{H}$,
one samples an independent SLE on $\mathbb{H}$. One then defines a process by opening up the
SLE and uniformizing the picture back to $\mathbb{H}$, while using a natural change of coordinate
for the free field to preserve the metric it represents. This unzipping process is stationary, as proven by Sheffield for SLE parameter $\kappa < 4$.
The Gaussian free field (GFF) conjecturally describes the scaling limit of discrete geometry. Typically, one studies the uniform measure on a certain class of large planar graphs equipped with their graph metric.
It is possible to decorate these graphs by drawing statistical mechanics models on them,
and thus reweighing the measure on metric spaces by the partition functions of a statistical mechanics model. The scaling limit of these decorated graphs can be conjecturally
described as a random metric space determined by a GFF, that moreover carries interfaces
of a model of statistical mechanics. In this way, SLE curves naturally appear in a coupling
with a GFF. In certain discrete settings, one can find a measure-preserving operation that
consists in cutting and opening up the graph alongside an interface of the statistical model
drawn on it. This discrete cutting process conjecturally converges to a continuous stationary process that we now describe. Given a Neumann free field in the upper half plane $\mathbb{H}$,
one samples an independent SLE on $\mathbb{H}$. One then defines a process by opening up the
SLE and uniformizing the picture back to $\mathbb{H}$, while using a natural change of coordinate
for the free field to preserve the metric it represents. This unzipping process is stationary, as proven by Sheffield for SLE parameter $\kappa < 4$.
One can use this process to construct a measure on the SLE by pushing the natural random boundary measure from $\mathbb{R}$ to the SLE via the zipping up operation. We provide an alternative description of this measure (called quantum time by Sheffield): we show that this quantum time can be seen as a chaos on the natural parametrization of SLE. In particular, this provides another construction of the natural parametrization of SLE for $\kappa < 4$.
 Ann. Inst. H. Poincaré Probab. Statist. 52, no. 4, 1784-1798
Ann. Inst. H. Poincaré Probab. Statist. 52, no. 4, 1784-1798
The Ising model is a magnetization model. Faces of the square lattice are fairly colored in black and white (or $+$ and $-$), and the law of the resulting coloring is then biased by a factor $e^{-2\beta}$ for each pair of neighboring faces whose colors disagree. For low values of the inverse temperature $\beta>0$, the picture is monochromatic in the scaling limit. For high values of $\beta$, the pictures is disordered at the microscopic level. However, there is a unique choice of a critical parameter $\beta_c$, where the Ising model exhibits a different behavior.
We prove that crossing probabilities for the critical planar Ising model with free boundary conditions are conformally invariant in the scaling limit. We do so by establishing the convergence of any interface joining two boundary points towards an SLE$_3(-3/2,-3/2)$. We also construct the joint limit of all Ising interfaces that touch the free boundary. They can be understood as forming an exploration tree analogous to the ones introduced by Sheffield.
 Ann. Inst. H. Poincaré Probab. Statist. 52, no. 3, 1406-1436
Ann. Inst. H. Poincaré Probab. Statist. 52, no. 3, 1406-1436
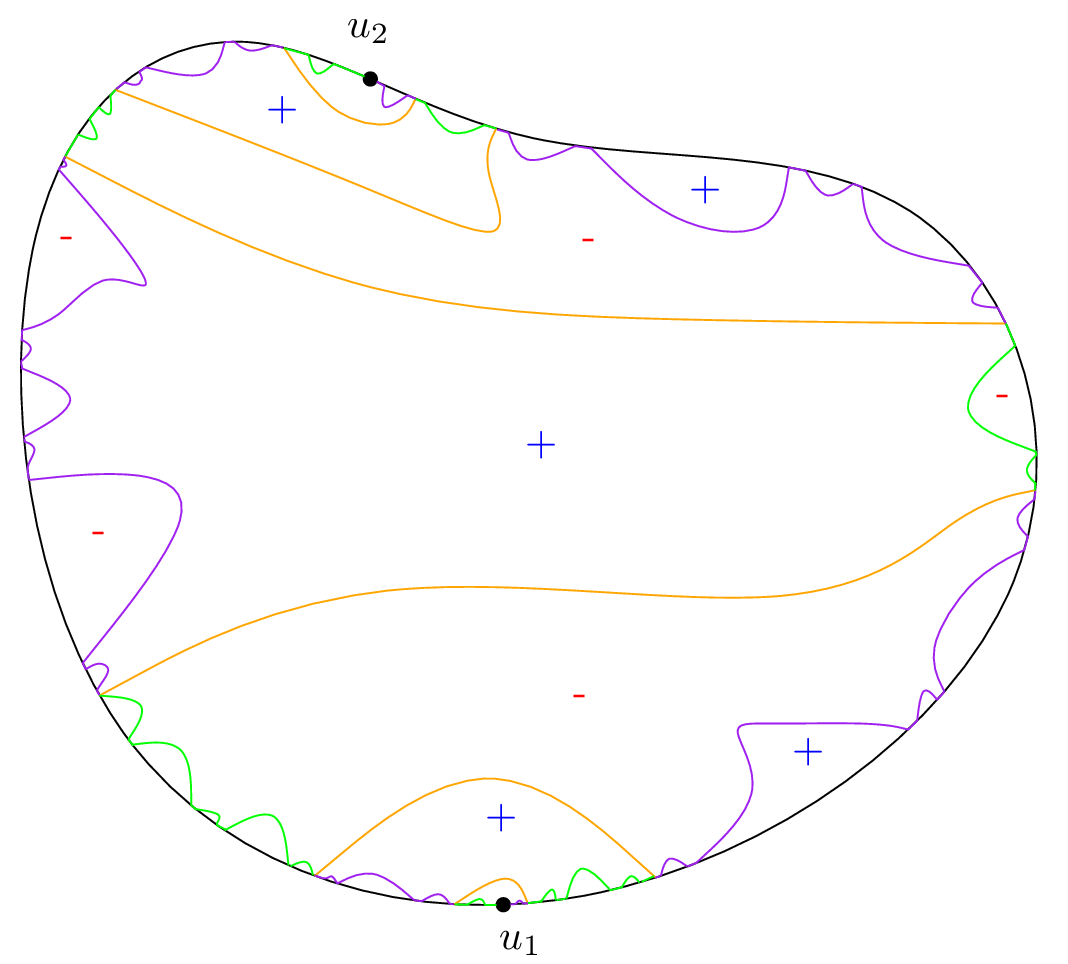
We are interested in random loops that arise as the scaling limits of statistical mechanics interfaces. Typically, one cuts up a domain of the plane by a lattice of very small mesh size, and randomly colors in black and white the lattice faces according to a certain law. The objects of interest are interfaces i.e. path that separate black and white regions. Such loops are expected to be conformally invariant in the scaling limit. Moreover, one may be able to explicitly compute how these random loops vary when the boundary of the domain is perturbed. Conjecturally, any such loop measure fall in a one parameter family indexed by the central charge. In particular, conjecturally, random curves are characterized by their covariance property, i.e. by their interaction with the boundary.
Werner showed that there is a unique way to associate to any Riemann surface a measure on its simple loops, such that the collection of measures satisfy a strong conformal invariance property (no interaction with the boundary). These random loops are constructed as the boundary of Brownian loops, and correspond in the zoo of statistical mechanics models to central charge $0$, or Schramm-Loewner Evolution (SLE) parameter $\kappa=8/3$.
The goal of this paper is to construct a family of measures on simple loops on Riemann surfaces that satisfies a conformal covariance property, and that would correspond to SLE parameter $\kappa=2$ (central charge $-2$).
 We study random two-dimensional spanning forests in the plane that can be viewed both in the discrete case and in their scaling limit as slight perturbations of
an uniformly chosen spanning tree. We show how to relate this scaling limit to a stationary distribution of a natural Markov process on a state of abstract
graphs with non-constant edge-weights. This simple Markov process can be viewed as a renormalization flow, so that in this two-dimensional case, one can give a rigorous meaning to the fact
that there is a unique fixed point (i.e. stationary distribution) in two dimensions for this renormalization flow, and when starting from any two-dimensional lattice, the renormalization flow (i.e. the Markov process)
converges to this fixed point (i.e. converges in law to its stationary distribution).
We study random two-dimensional spanning forests in the plane that can be viewed both in the discrete case and in their scaling limit as slight perturbations of
an uniformly chosen spanning tree. We show how to relate this scaling limit to a stationary distribution of a natural Markov process on a state of abstract
graphs with non-constant edge-weights. This simple Markov process can be viewed as a renormalization flow, so that in this two-dimensional case, one can give a rigorous meaning to the fact
that there is a unique fixed point (i.e. stationary distribution) in two dimensions for this renormalization flow, and when starting from any two-dimensional lattice, the renormalization flow (i.e. the Markov process)
converges to this fixed point (i.e. converges in law to its stationary distribution).
While the results of this paper are dealing with the planar case and build on the convergence in distribution of branches of the UST to SLE$_2$ as well as on the predicted convergence of the suitably renormalized length of the loop-erased random walk to the ''natural parametrization'' of the SLE$_2$, this Markov process setup is in fact not restricted to two dimensions.
 Given a $\mathcal{C}^1$ simple loop in the plane, we define a family of Markov processes $(X^p_t)_{p\in [0,1]}$ that interpolates between reflected Brownian Motion inside ($p=0$) and outside ($p=1$) of the curve $\ell$, via usual Brownian Motion ($p=1/2$). We construct these processes by gluing Brownian excursions from $\ell$ to itself. A coin biased according to the interpolation parameter $p$ is thrown for each excursion in order to decide whether it will be glued inside or outside the curve $\ell$.
Given a $\mathcal{C}^1$ simple loop in the plane, we define a family of Markov processes $(X^p_t)_{p\in [0,1]}$ that interpolates between reflected Brownian Motion inside ($p=0$) and outside ($p=1$) of the curve $\ell$, via usual Brownian Motion ($p=1/2$). We construct these processes by gluing Brownian excursions from $\ell$ to itself. A coin biased according to the interpolation parameter $p$ is thrown for each excursion in order to decide whether it will be glued inside or outside the curve $\ell$.
A related question (not considered in this paper) is to understand if one can do the same when the loop $\ell$ is only continuous (and what about when $\ell$ is the boundary of a simply-connected domain ?). Even in the most irregular cases, Brownian Motion and reflected Brownian Motion are well-defined. Can one understand the relation between Brownian Motion and its excursions off the loop $\ell$ ?
Answering these questions could be the first step towards building an interpolation between Brownian Motion and SLE$_6$. Indeed, notice that SLE$_6$ is the scaling limit of the percolation interface, which is nothing else than a random walk conditioned on never crossing its past. In this sense, SLE$_6$ itself can be thought of as a Brownian Motion reflected off its past.




