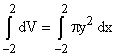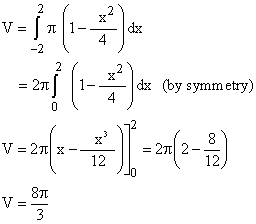Calculate the volume generate by rotating the ellipse of equation around the x-axis.

Introduction

The method of disks consists of slicing the figure in question into disk shaped slices, computing the volume of each and summing, ie, integrating over these.

Comment

Rotate the ellipse

By rotating the ellipse around the x-axis,  we generate a solid of revolution called an ellipsoid whose volume can be calculated using the disk method.

Disk method

We revolve around the x-axis a thin vertical strip of height y = f(x) and thickness dx.

This generates a disk of radius y and thickness dx whose volume is dV.

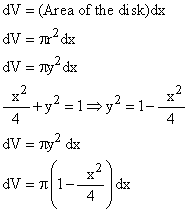
Volume of the ellipsoid

We get the volume of the ellipsoid by filling it with a very large number of very thin disks, that is by integrating dV from x = -2 to x = 2.

Volume of th  ellipsoid = V =