![]()
18.325
Vortex Dynamics
MIT
Department of Mathematics
Spring term 2006
TR: 1pm-2.30pm in 2-103
![]()
This advanced course will give an introduction to vortex dynamics aimed at applied mathematicians. Only a basic knowledge of fluid dynamics will be assumed. Mathematical prerequisites are a knowledge of calculus, vector calculus and complex analysis.
Take a look at a review article on "Point vortex motion" that will appear in the upcoming "Encyclopedia of Mathematical Physics". It provides an accessible introduction to the key ideas underlying vortex dynamics.
Some of the topics to be covered:
-
concept of vorticity; the Biot-Savart integral
-
point vortex dynamics; the Hamiltonian formulation; vortex collapse, leap-frogging
-
vortex motion around topography (Kirchhoff-Routh theory)
-
the vortex patch model; Kirchhoff ellipse; Moore-Saffman-Kida generalizations
-
theory of vortex interactions (the "elliptical vortex model");
-
vortex merger
-
vortex stability
-
contour dynamics; numerical methods
![]()

-
vortex rings; Hill's spherical vortex
-
vortex sheets; Birkhoff-Rott formulation
-
essentials of wing theory, aerodynamics
-
vortex motion on a spherical surface; geophysical and astrophysical applications
-
biological vortex dynamics (propulsion of organisms)
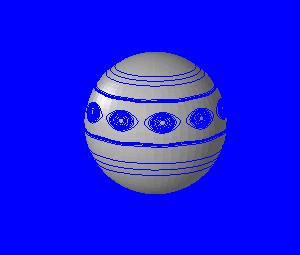
This
figure shows new analytical solutions
for "Stuart
vortex
layers" on a spherical surface
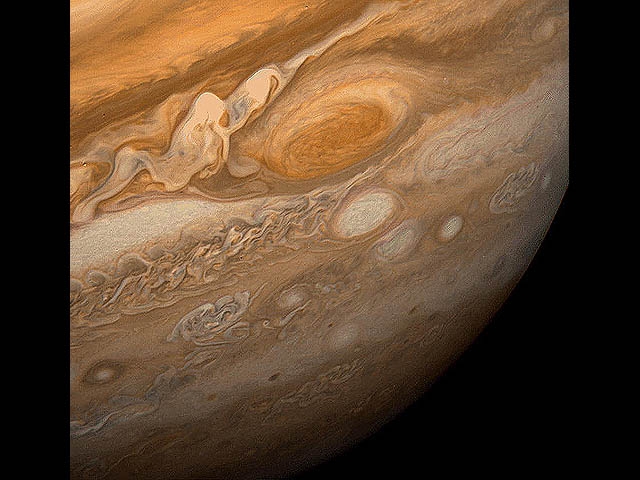
Photographs
of vortical layers in the Jovian atmosphere
(Courtesy
of NASA)