There are two directions: the r direction is the direction of a vector from the origin to the point in question;
a unit  vector in this direction has representation:

u_r = i cos+ j sin

thedirection is normal to this:

u = -i sin + j cos

The vector r is represented in this coordinate system by r = ru_r , where r = (x²+y²)^1/2;
since we have

r(t) = x(t) i + y(t) j

we obtain

x = rcos , y = rsin

Taking derivatives we find

verify by differentiating yourself that

which gives:

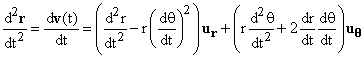
The second and fourth terms here are sometimes referred to in physics as the centrifugal and Coriolis forces. Thus if an object is subject to no external force, so that

you will find that it obeys

,

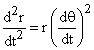and

The former causes the radial velocity to grow if there is angular motion: the latter slows down the angular motion if the object is moving away from the origin.

Polar - cartesian coordinates converter