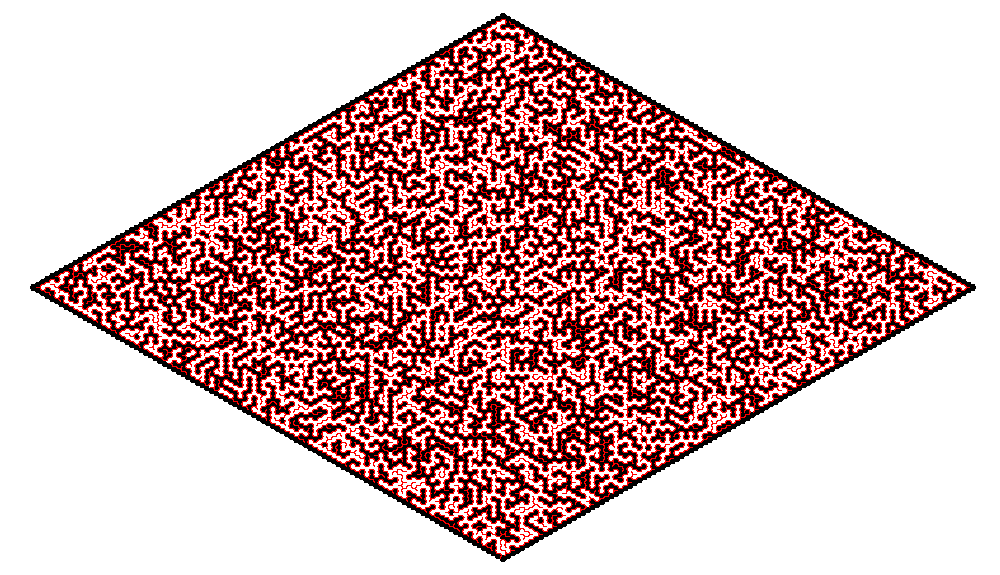
Conformal loop ensembles:
The conformal loop ensembles CLE(kappa), indexed by a parameter kappa
between 8/3 and 8, are random collections of countably many disjoint loops
in a planar domain. When kappa is less then or equal to 4, the loops are
almost surely simple and disjoint. For larger kappa, the loops may
intersect themselves and one another. An instance of CLE(8/3) is almost
surely empty (i.e., no macroscopic loops) . An instance of CLE(8) is
almost surely a single space filling loop. There is a natural coupling
between a CLE on a planar domain and the Gaussian free field on that
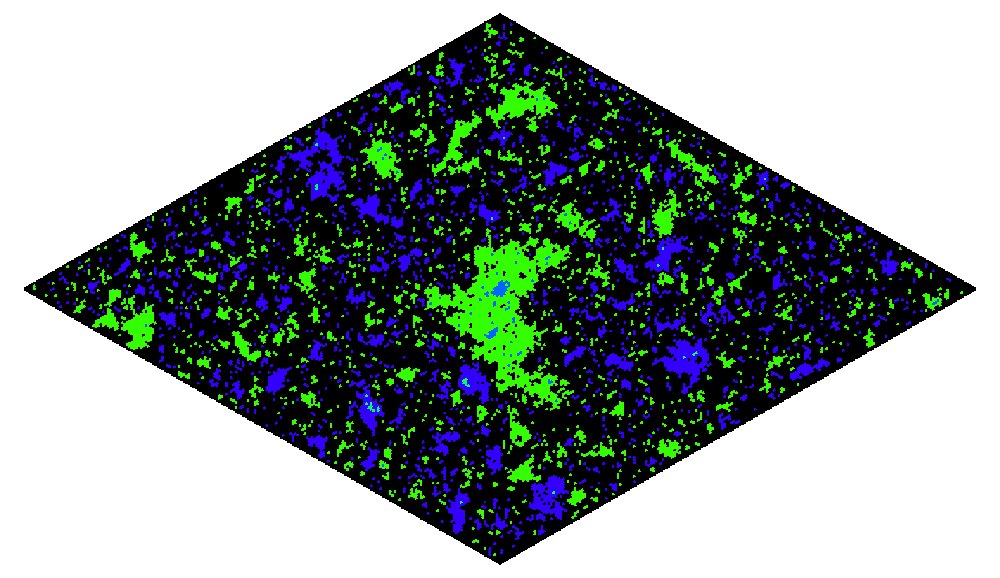
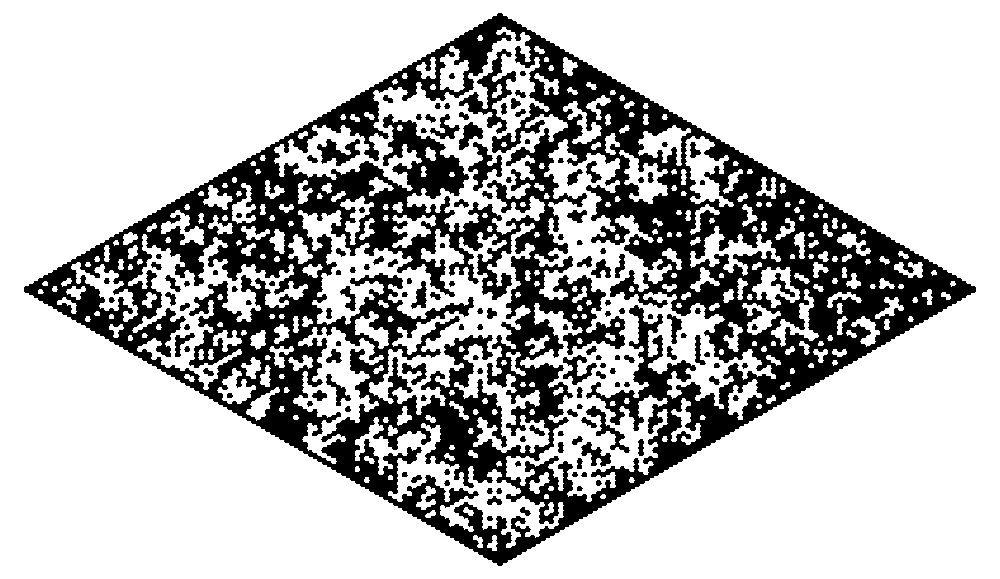
domain (which I don't describe here). The boundaries between black
and white clusters in the figures below are discrete approximations to the
conformal loop ensembles constructed from the discrete Gaussian free
field.
- CLE(3)
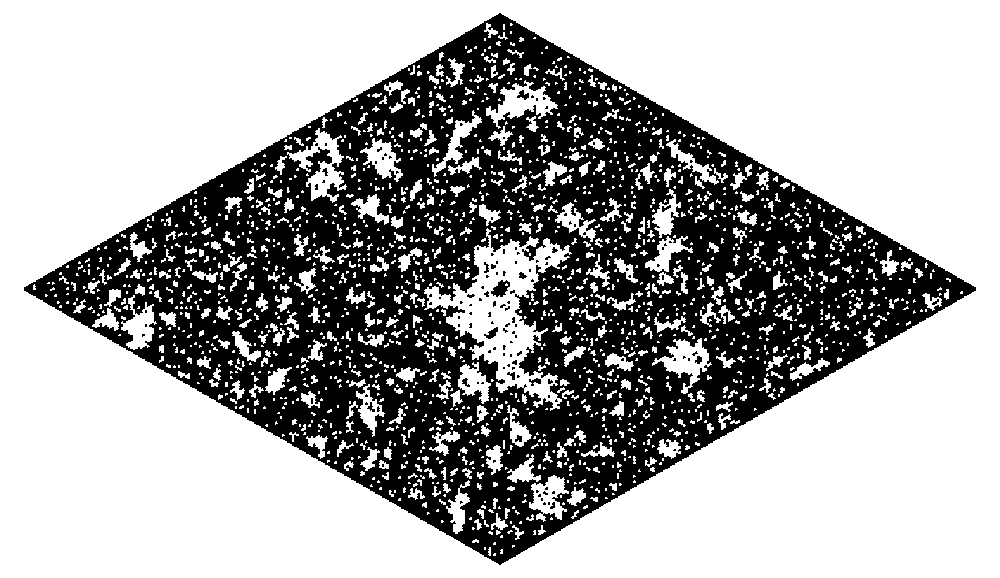
- CLE(3) in color
- CLE(4.5)
- CLE(4.5) loops that hit boundary
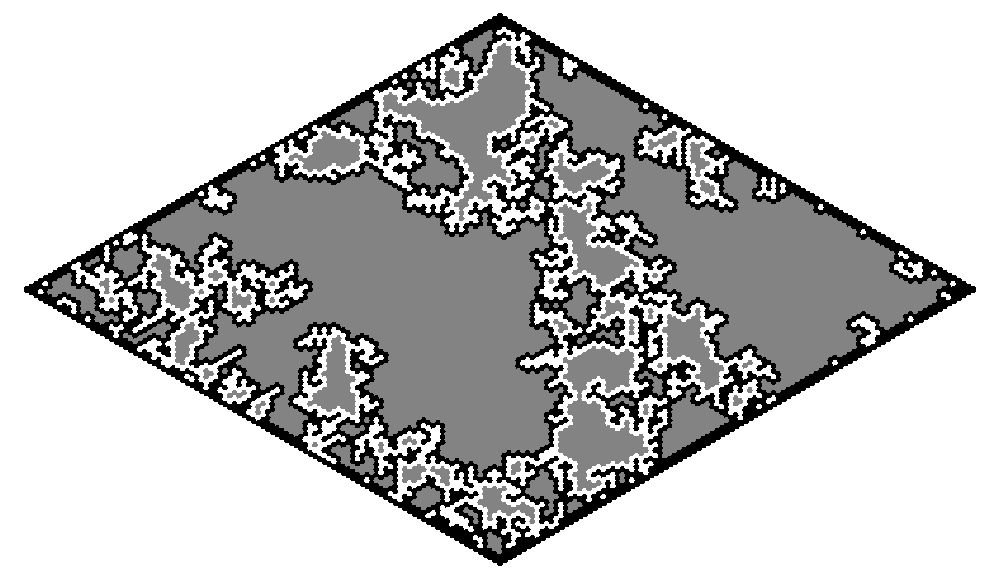
- CLE(4.5) with exploration tree
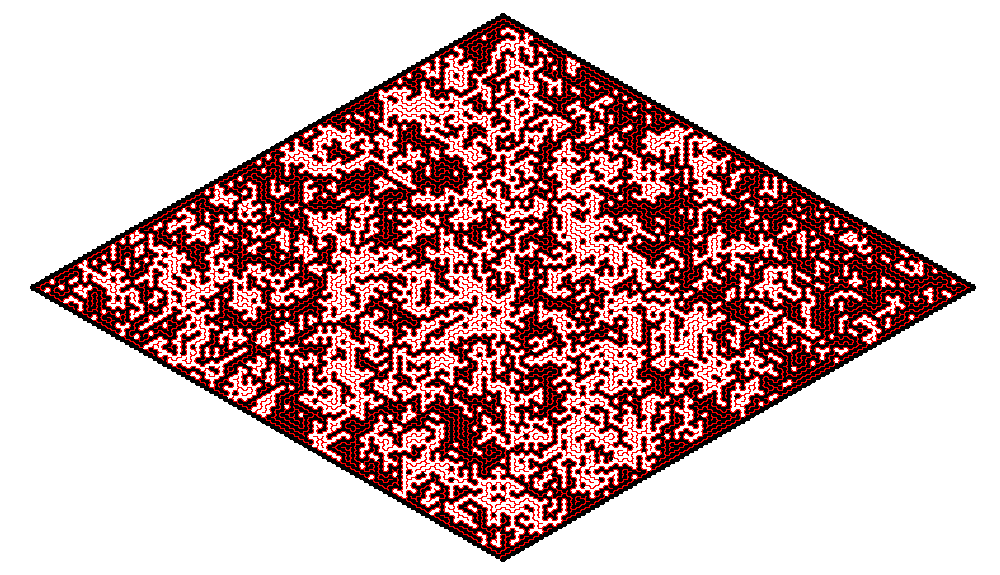
- CLE(7)
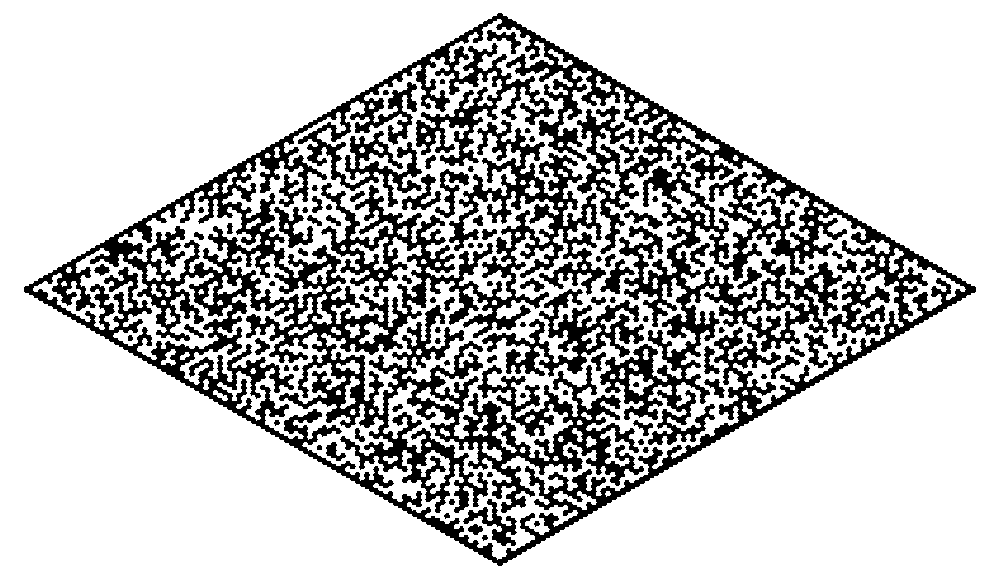
- CLE(7) loops that hit boundary
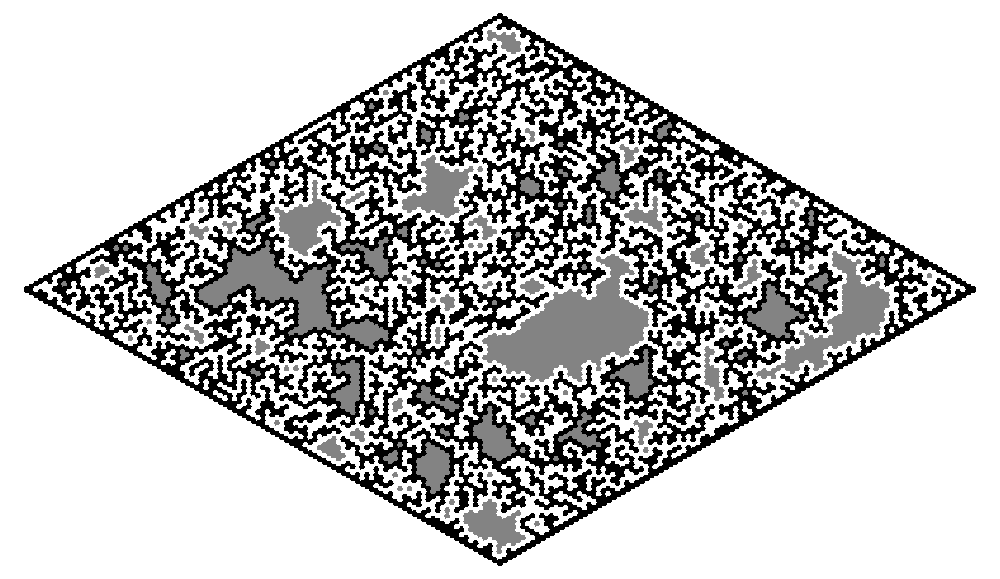
- CLE(7) with exploration tree