Practice test 1

(Also available as postscript and acrobat file off my web page).

The test on Thursday will be open book - just the book, nothing else is permitted (and no notes in your book!) Note that where $\mathbb{R}^k$ is mentioned below the standard metric is assumed.

Let $C\subset\mathbb{R}^n$ be closed. Show that there is a point $p\in C$ such that $\vert p\vert=\inf\{\vert x\vert;x\in X\}.$
Give a counterexample to each of the following statements:
1. Subsets of $\mathbb{R}$ are either open or closed
2. A closed and bounded subset of a metric space is compact.
3. In any metric space the complement of a connected set is connected.
4. Given a sequence in a metric space, if every subsequence of that sequence itself has a convergent subsequence then the original sequence converges.
Suppose and are connected subsets of a metric space 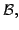and that $A\cap B\not=\emptyset,$ show that $A\cup B$ is connected.
Let $i=1,\dots,N,$ be a finite number of compact sets in a metric space Show that $\bigcup_{i=1}^NK_i$ is compact.
Let $G_i\subset X,$ $i\in\mathbb{N}$ be a countable collection of open subsets of a complete metric space, Suppose that for each $N\in\mathbb{N},$ $\bigcap_{i=1}^NG_i\not=X$ and that for each $x,y\in X\setminus G_n\Longrightarrow d(x,y)<1/n.$ Show that $\bigcup_{i\in\mathbb{N}}G_i\not=X.$
Let be a bounded sequence in $\mathbb{R}.$ Show that there exists $x\in\mathbb{R}$ and a subsequence $x_{n(k)}$ such that $\sum\limits_{k=1}^\infty x_{n(k)}-x$ converges absolutely.

Richard B. Melrose 2004-05-18