These genus 2 curves have simple Jacobians that are either geometrically simple abelian surfaces with real multiplication (defined over a quadratic extension) or isogenous to the restriction of scalars of an elliptic curve defined over a qudaratic field.
Each blue/red dot at (p,ap) records the average of ap(X) over genus 2 curves X/Q with Sato-Tate group N(SU(2)xSU(2)) whose L-functions have root number w(X)=+1/-1 with conductor in a varying dyadic interval.
 |
Each purple dot at (p,ap) records the average of w(X)ap(X) over genus 2 curves X/Q with Sato-Tate group N(SU(2)xSU(2)) and conductor in a varying dyadic interval.
 |
Each purple dot at (p,ap) records the average of w(X)ap(X) over genus 2 curves X/Q with Sato-Tate group N(SU(2)xSU(2)) and conductor in a varying dyadic interval. The green line shows a least squares fit using a piecewise polynomial function.
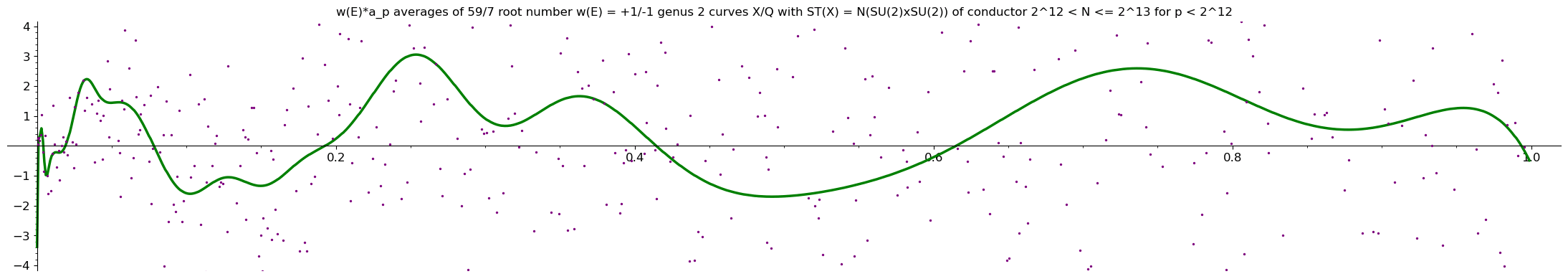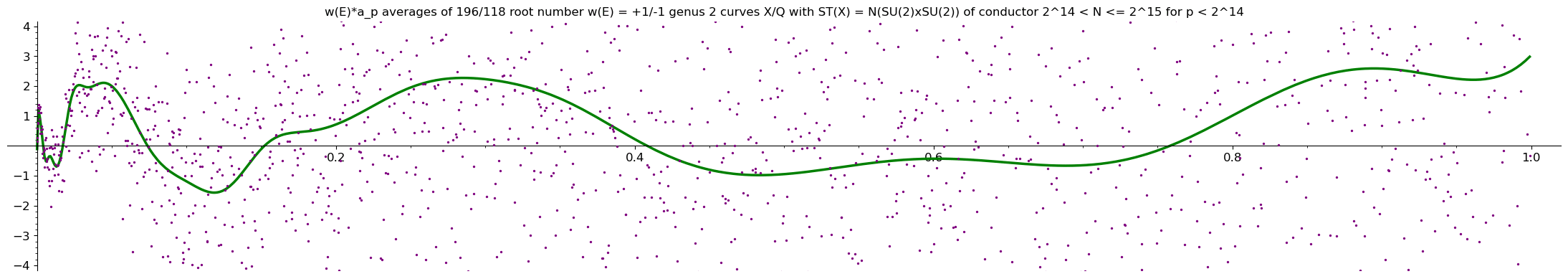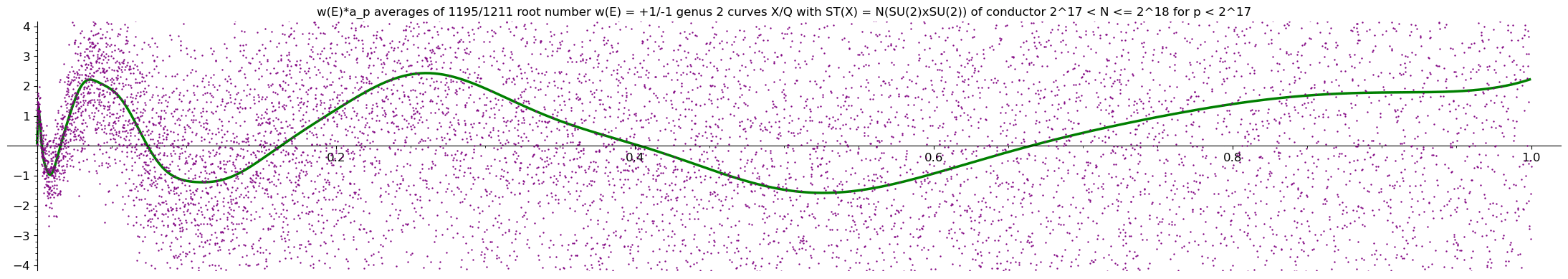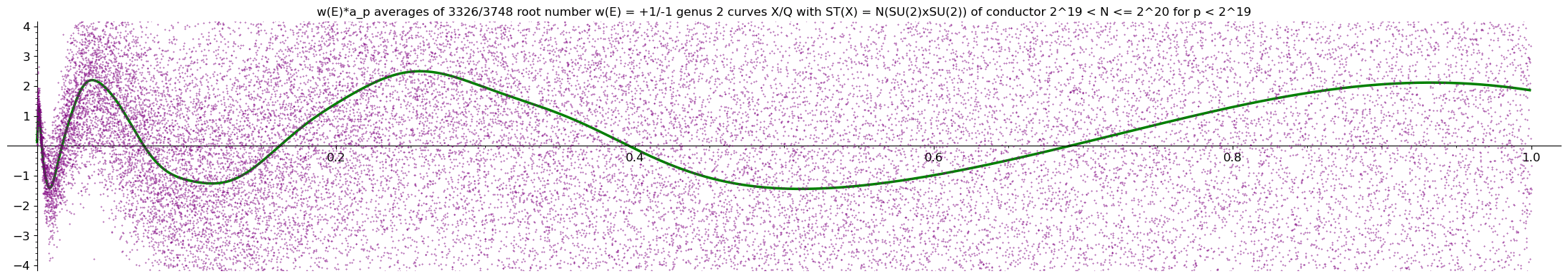 |