
Each blue/red dot at (p,ap) records the average of ap(A) over abelian surfaces isogenous to a product of non-CM elliptic curves over Q whose L-functions have root number w(A)=+1/-1 with the conductor of A in a varying dyadic interval.
 |
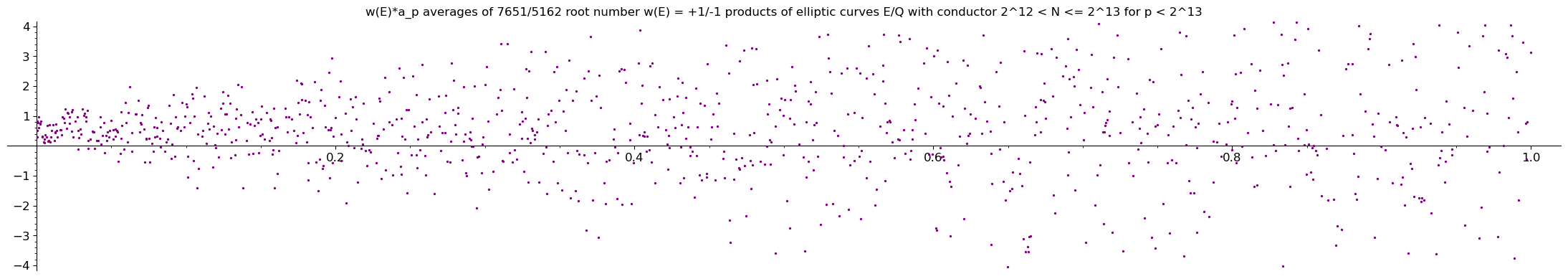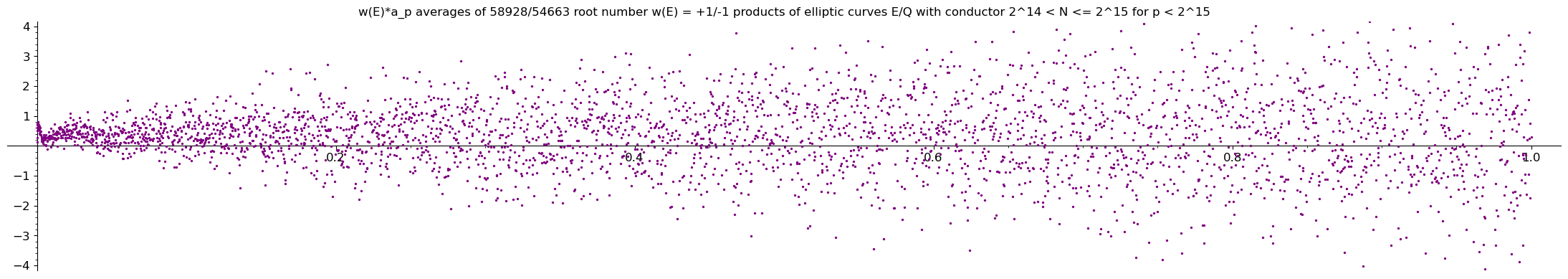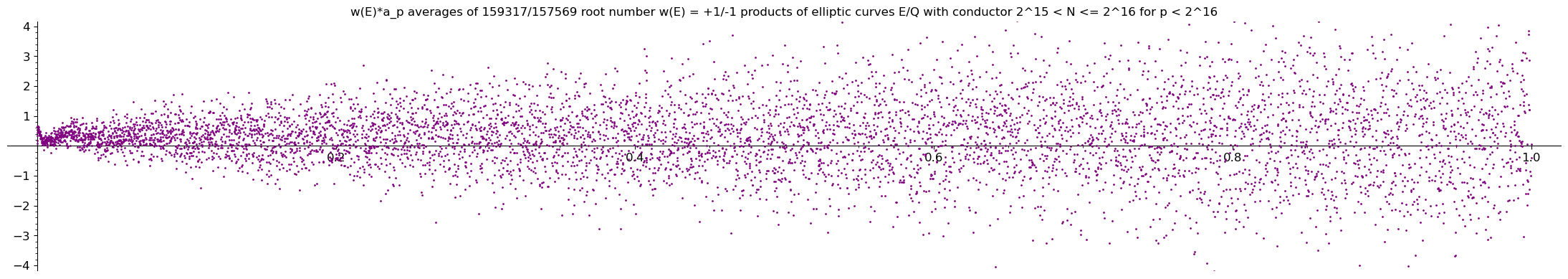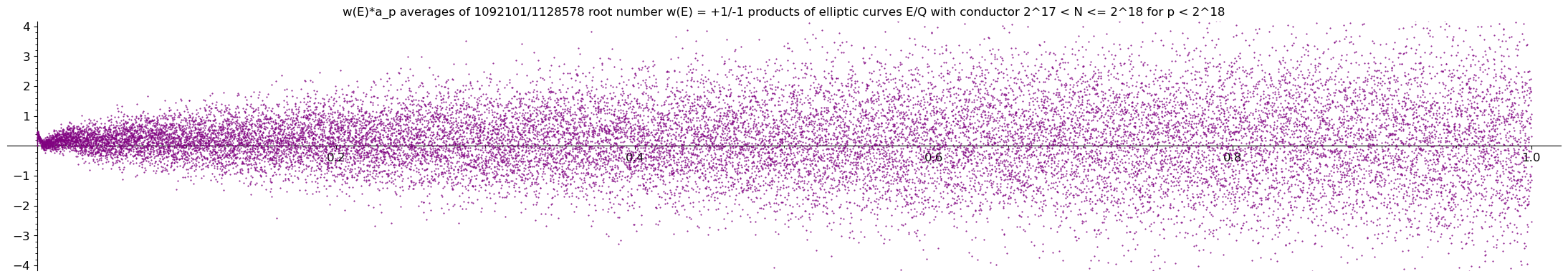
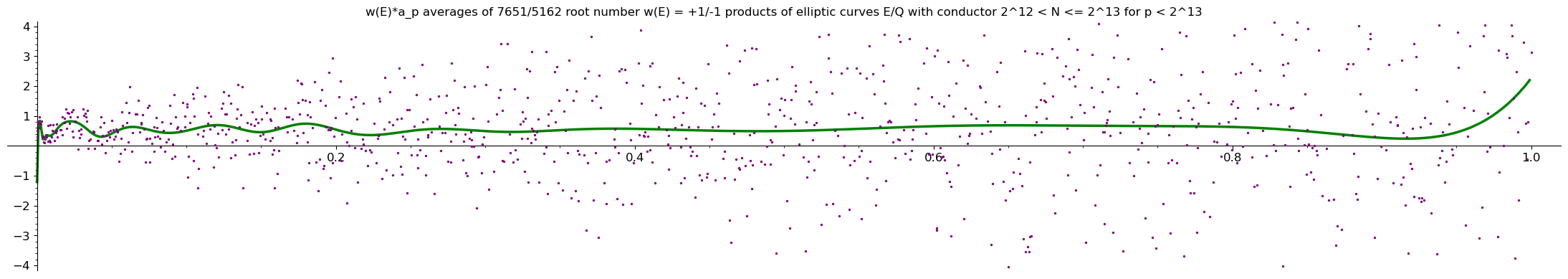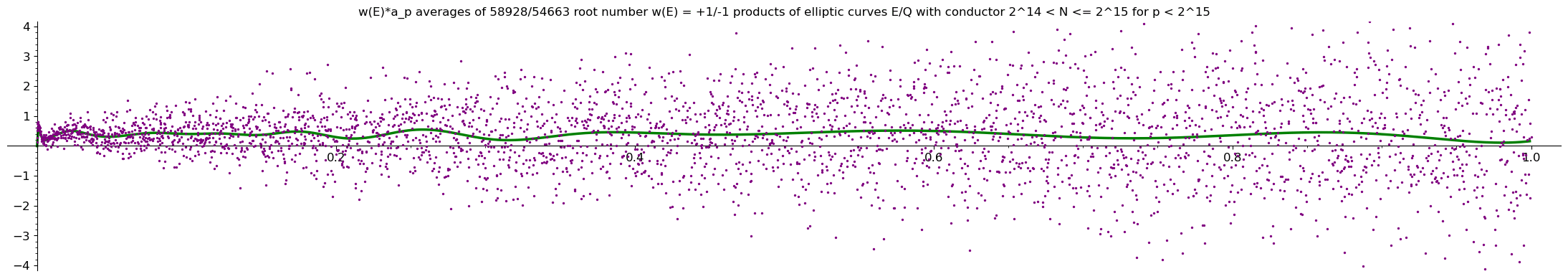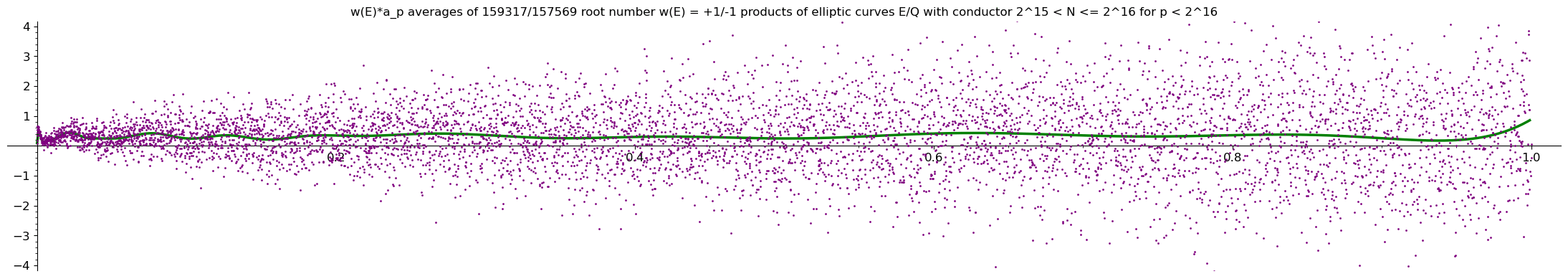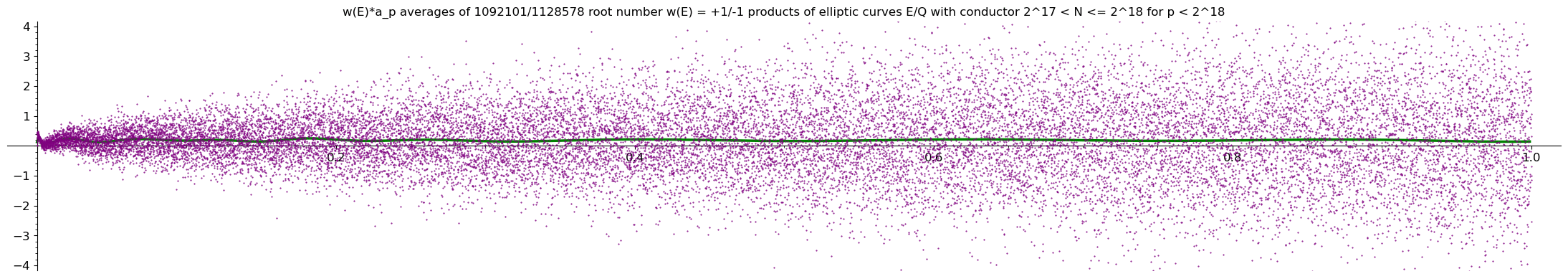
Each purple dot at (p,ap) records the average of w(A)ap(A) over abelian surfaces isogenous to a product of non-CM elliptic curves with the conductor of A in a varying dyadic interval.
 |
Each purple dot at (p,ap) records the average of w(A)ap(A) over abelian surfaces isogenous to a product of non-CM elliptic curves with the conductor of A in a varying dyadic interval. The green line shows a least squares fit using a piecewise polynomial function.
 |