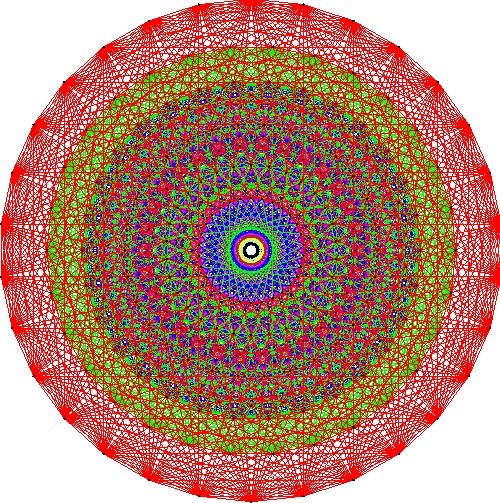
This is a picture of the root system of type E8, projected from 8 dimensions down to 2. Picture by John Stembridge, based on a drawing by Peter McMullen.
In eight dimensions each root is a vector of the same length (often chosen to be the square root of 2). In this projection, some of the vectors are shorter. In fact there are 30 vectors of the greatest possible length (the small dots on the outer circle), 30 of the next greatest length, and so on. There may appear to be lots more than eight circles of dots; many of the smaller ones are visual artifacts of intersecting lines in the drawing.
Each root is connected by a line to its 56 nearest neighbors, each of which is at distance square root of two; so two adjacent roots and the origin make an equilateral triangle in eight dimensions. After projection to two dimensions, pairs of these 56 lines coincide, so you see only 28 lines coming out of each root image. The picture does not show lines from the origin to each root.
The configuration in eight dimensions is called the Gossett polytope 421. You can read all about it and see many more intricately beautiful pictures in the book "Regular complex polytopes" by H.S.M. Coxeter. (And you thought I was going to give you another link! Naw, I'm the resident dignified older individual).
John Stembridge has a web page with a more detailed and complete technical explanation of the picture, along with a number of simpler examples. He has also provided eps files from which you can print arbitrarily high-resolution versions of the pictures. He and I have slightly different aesthetic tastes (wrong and right, to oversimplify a bit), so I will provide here a small modification of his high-resolution image of the E8 root system.