Couplings of SLE and Gaussian free field:
There is a way to couple the Schramm-Loewner evolution SLE(kappa)
with the Gaussian free field so that conditioned on the path,
the expected height of the field near the path is determined by the
winding of the path (see the Fields Institute
lectures for a precise explanation) .
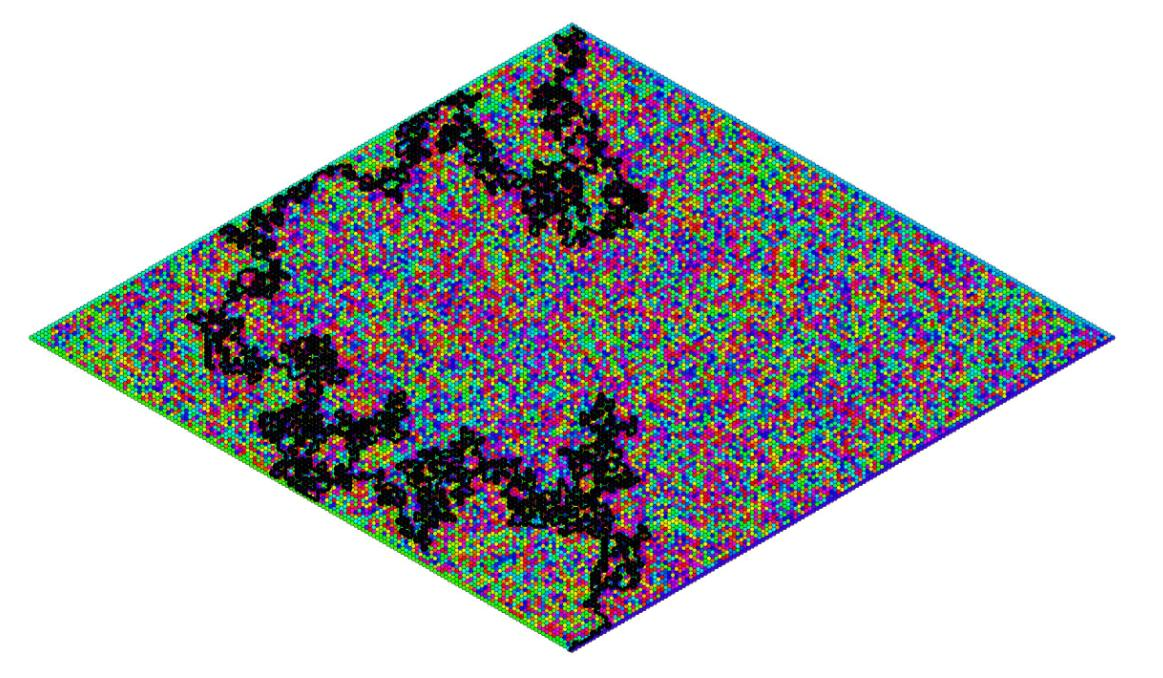
The following figures represent discrete analogs of this coupling. To
draw such a figure, one first samples a
discrete Gaussian
free field with
appropriate boundary conditions---this gives us a random real-valued
function h on the
hexagonal faces (which are colored according to the function values).
The black paths are then generated dynamically from bottom to top. When
the path
hits a hexagon it has not visited before, it decides whether to turn left
or right by comparing a constant multiple of the winding of the path
(number of right minus number of left turns thus far) with the value
of h on that
hexagon. Different constants correspond to
different values of kappa.
When the constant is zero, the winding is irrelevant, and the path
always turns right when h is negative and left when it is
positive. In this case, the path is a boundary between clusters of
positively and negatively valued hexagons. This case corresponds to kappa = 4
and is studied in detail in joint work with
Oded Schramm.
A figure similar to those below appeared in
Scientific American .
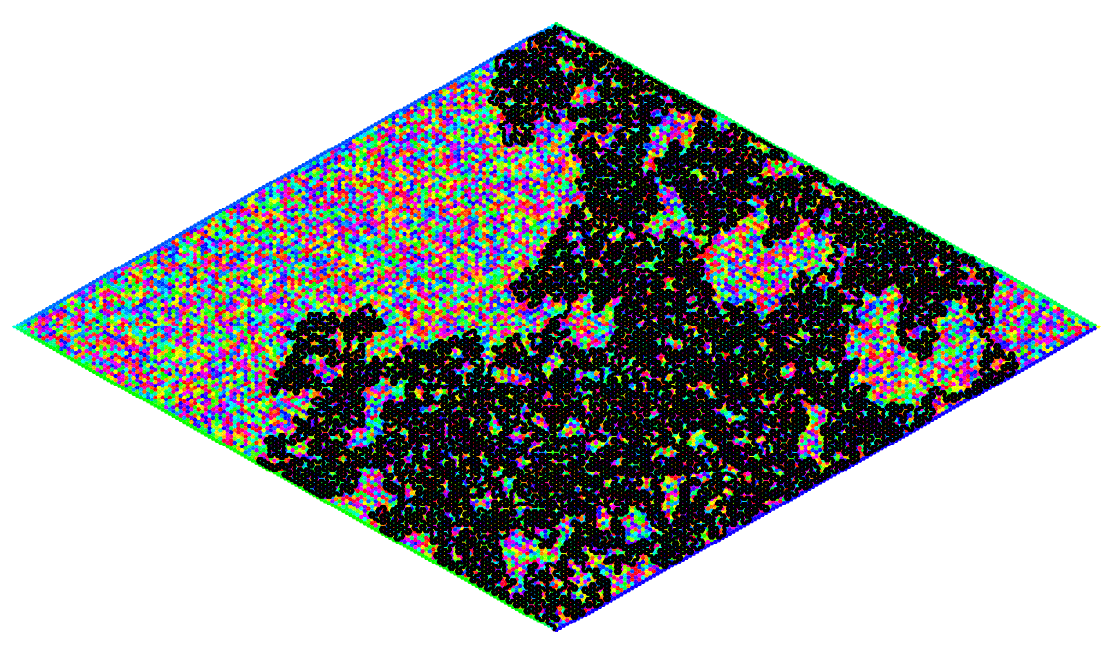
- SLE(.5)
- SLE(8/3)

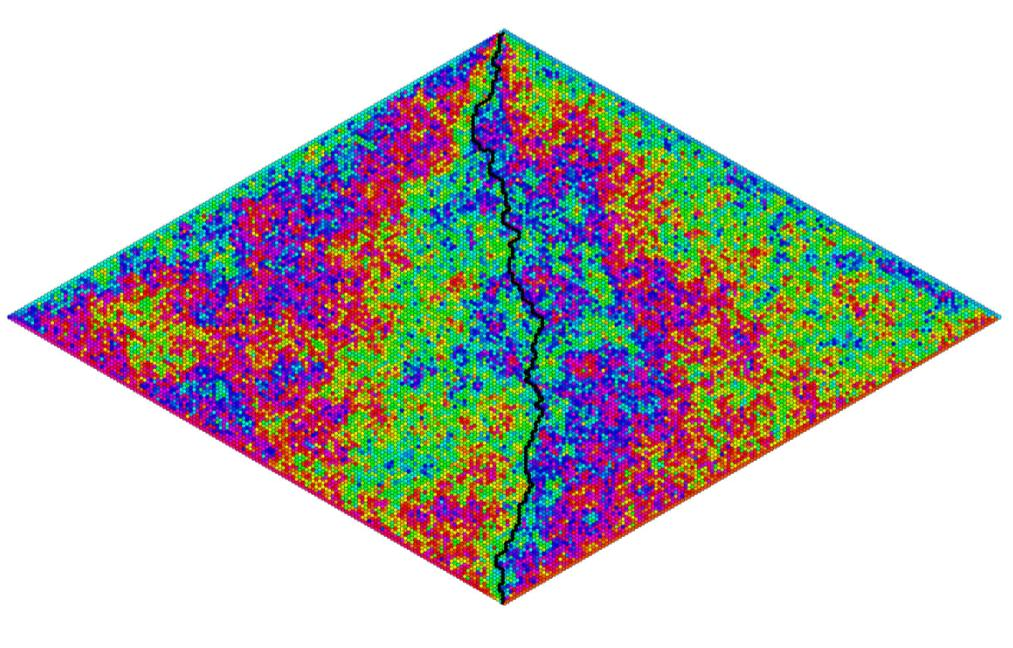
- SLE(3)
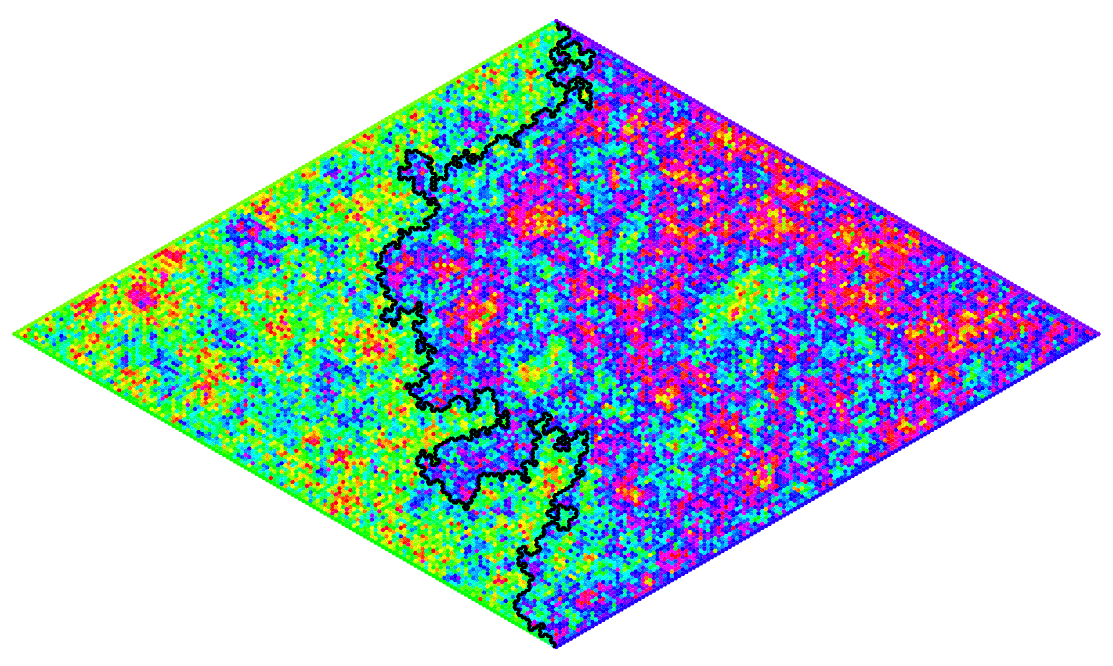
- SLE(4)
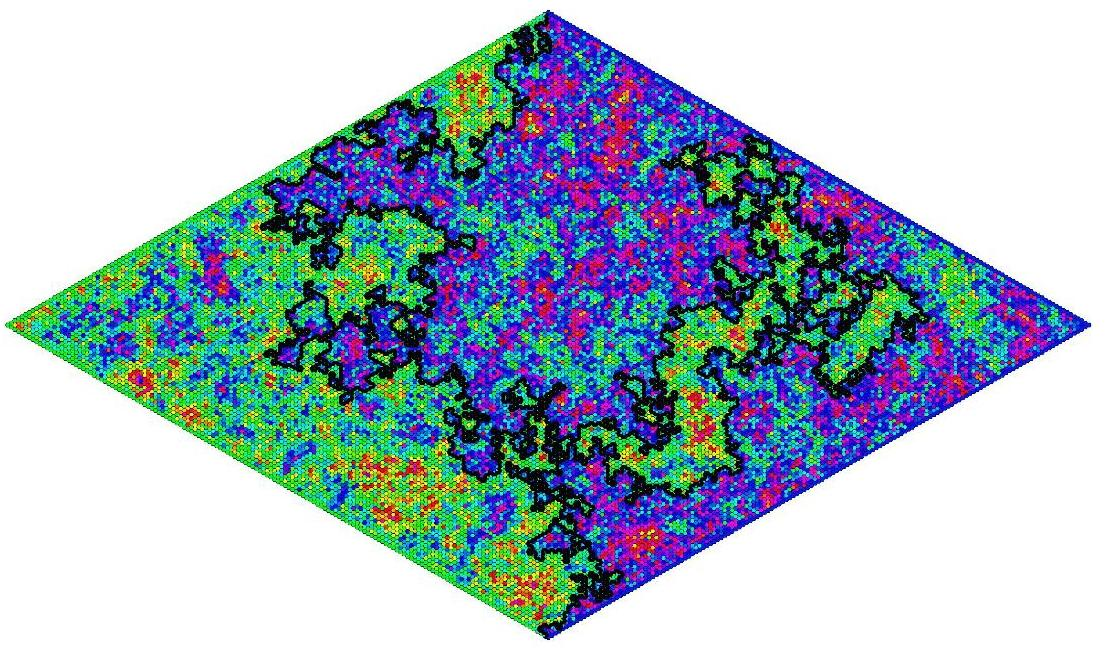
- SLE(6)
- SLE(7.5)