18.103, Spring 2004

Richard Melrose

Department of Mathematics, Massachusetts Institute of Technology

rbm@math.mit.edu

Tuesday and Thursdays, 1-2:30 in Room 4-102. The book is Adams and Guillemin [1]

Lecture 1:

February 3.

Read A-G, Chapter 1, pages 1-4, 24-26.

Problems, A-G, Chapter 1, p.11, Problems 1, 2; p;39 Problems 1 (first part), 2 Here are the problems

1.1.1. Prove that the set $\mathcal{B}$ of Bernoulli sequences is uncountable by the Cantor diagonal argument.

1.1.2. a) Let $\omega \in I=(0,1].$ Show that $\omega$ can be written in the form $\sum\limits_{i=1}^\infty \frac{a_i}{2^i}$ with $a_i=0$ or $1.$ Show that this expansion is unique when we restrict to nonterminating series.

b) Show that for any (positive) integer $k,$ $\omega \in I$ can be written in the form $\sum\limits_{i=1}^\infty \frac{a_i}{k^i}$ where $a_i=0,1,\dots,k-1.$ Show that the expansion is unique when we restrict to nonterminating series.

1.3.1. Let $X$ be an uncountable set. Let $\mathcal{R}$ be the collection of all finite subsets of $X.$ Given $A\in\mathcal{R},$ let $\mu(A)$ be the number of elements in $A.$ Show that $\mathcal{R}$ is a ring and that $\mu$ is a measure on $\mathcal{R}.$

1.3.2. Let $X$ be an infinite set and let $\mathcal{R}$ be the collection of sets consisting of the finte sets and the complements, in $X,$ of finte sets. Let $\mu$ be the function $\mu(A)=0$ if $A$ is finite, $\mu(A)=1$ if the complement of $A$ is finite. Is $\mu$ a measure?

Lecture 2:

February 5.

Read A-G, pp 27-31, pp 4-9

Problems: p.39, nos. 3, 4; p.12 nos. 4,5. Here are the problems

1.3.3. a) Let $X$ be an infinte set and $\mathcal{R}$ the collection of all countable subsets of $X.$ Is $\mathcal{R}$ a ring?

b) Let $\mu$ be a measure on $\mathcal{R}.$ Show that there exists a function $f:X\longrightarrow [0,\infty)$ such that

$$\mu(A)=\sum\limits_{x\in A}f(x)\ \forall\ A\in\mathcal{R}.$$ (1)

c) Show that the funcion $f$ in part b) has the following two properties. (1) the set $\{x\in X;f(x)\not=0\}$ is countable and (2) $\sum\limits_{x\in X}f(x)<\infty.$

d) Show that if $f$ has the properties in part c) the formula (1) defies a measure on $\mathcal{R}.$

1.3.4. Let $X$ be the real line and $\mathcal{R}=\mathcal{R}_{\text{Leb}}.$ Given $A\in\mathcal{R}$ let $\mu(A)=1$ if, for some positive $\epsilon,$ $A$ contains the interval $(0,\epsilon)$ and otherwise $\mu(A)=0.$ Show that $A$ is an additive set function but is not countably additive.

1.1.4. Show that

$$\int_0^1 R_{\gamma _1}R_{\gamma _2}\cdots R_{\gamma_n}dx=0 1$$

for any sequence $\gamma _1\le\gamma _2\le\cdots\le\gamma _n.$ When is it $1?$

1.1.5. Define the Rademacher functions on the whole real line by requiring them to be periodice of period one - so setting $R_k(x+1)=R_k(x).$ With this definition show that $R_{k+1}(x)=R_k(2x)$ and by induction, $R_k(x)=R_1(2^{k-1}x).$

Countable additivity and subadditivity

Outer measure

Sets of measure zero

Measurable sets

Rademacher functions

Lecture 3:

February 10.

(Special) Chebyshev's inequality

Weak law of large numbers

Lecture 4:

February 12.

Homework due Friday 20th: Chapter 1, p. 41, nos 11, 13; p. 42 nos 19, 20.

A-G, pp 33-39.

The $\sigma$-ring of measurable sets.

The law of large numbers.

Lecture 5:

February 19 (Monday schedule on February 17).

A-G pp 53-58.

Measurable functions

Lecture 6:

February 24. The integral

Countable additivity: Theorem 11.

Lecture 7:

February 26. Linearity of the integral.

Proof of Theorem 11 finished.

Monotone convergence.

Additivity for positive functions.

The space $L(\mu,\mathcal F)$ of integrable functions (on $X$ with respect to the measure $\mu$ on the $\sigma$-ring $\mathcal F.$

Linearity of the integrable on integrable functions.

Lecture 8:

March 2.

A-G pages 77-80.

Fatou's lemma (for a sequence of non-negative measurable functions

$$\int_E\liminf_n f_nd\mu\le \int_E\liminf_nd\mu.$$

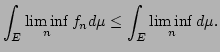
Lebesgue's Dominated convergence theorem. Pointwise convergence of a sequence of measurable functions with absolute value bounded by a fixed integrabe function implies convergence of the integrals to the integral of the limit.

The Banach space $L^1(X,\mu).$

Lecture 9:

March 4.

The Banach space $L^1(X,\mu)$ continued.

A Hilbert space, $L^2(X,\mu).$

Lecture

. 10:] March 9.

Hilbert spaces

Bessel's inequality

Existence of a complete orthonormal basis in a separable Hilbert space.

Lecture 11:

March 11.

Outline of proof that an $L^1$ function on $[-\pi,\pi]$ is determined by its Fourier coefficients.

Equality of Riemann and Lebesgue integrals of a continuous function on $[a,b].$

Convergence of the Fourier series of a function a differentiable function.

Lecture 12:

March 16.

Parallelogram law in a Hilbert space; converse explained but not done.

A non-emtpy closed convex set in Hilbert space has a unique point closest to the origin.

Linear functions on a normed space are continuous if and only if they are bounded.

Riesz Representation Theorem:- Any continuous linear functional on a Hilbert space is of the form $H\ni x\longmapsto \langle x,y\rangle$ for some $y\in H.$

Lecture 13:

March 18. First in-class test. Covers all material in Lectures 1-10.

Lecture 14:

March 30.

Lecture 15:

April 1. (I will be away and Professor Helgason will lecture)

Schwartz functions

Fourier transform

Inverse transform

Lecture 16:

April 6.

Metric on the space of Schwartz functions

Density of Scwhartz functions in $L^2(\mathbb{R}).$

Extension of Fourier transform to $L^2(\mathbb{R}).$

Lecture 17:

April 8.

Density of Schwartz functions in $L^1(\mathbb{R}).$

Harmonic oscillator, creation and annihilation operators.

Invariance under Fourier transform.

Completeness of eigenfunctions.

Weak solutions.

Lecture 18:

April 13.

Lecture 19:

April 15.

Lecture 20:

April 22 (April 20 is a holiday).

Lecture 21:

April 27. Second in-class test.

Lecture 22:

April 29.

Lecture 23:

May 4.

Lecture 24:

May 6.

Lecture 25:

May 11.

Lecture 26:

May 13.