A 3d representation for domino tilings of the Aztec diamond

Alexei Borodin and Matvey Borodin

Random domino tilings the Aztec diamonds, along with random lozenge tilings of a hexagon, is one of the most studied models of statistical physics. It was first introduced by Elkies-Kuperberg-Larsen-Propp in [3], and we refer to a recent survey by Johansson [4] and references therein for detailed information about it.

Here is an image of a domino tiling of the Aztec diamond of order 4.

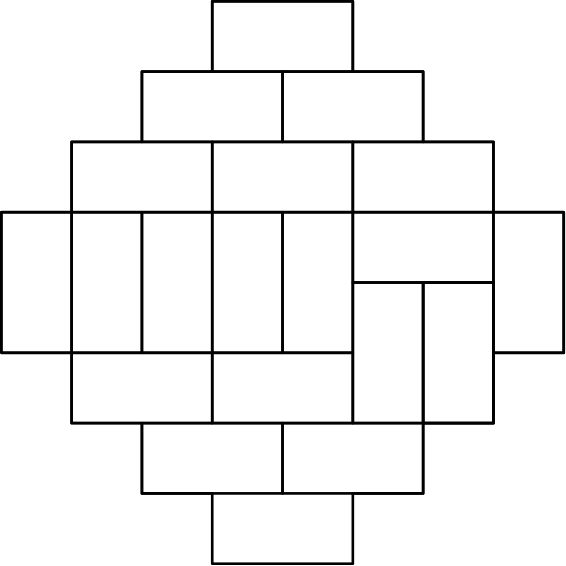
There are two types of dominoes in the picture - horizontal and vertical. However, if one paints the grid in the checkerboard manner, then there will be four types of dominoes:

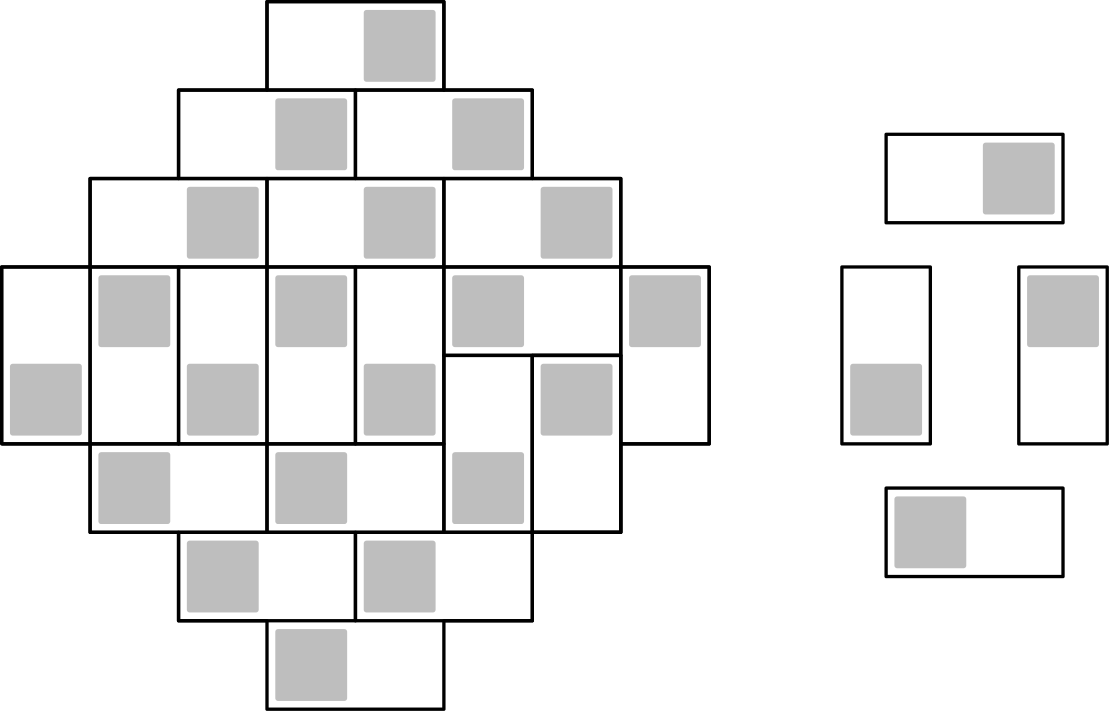
A more visual way to encode the four different types of dominoes is to paint them in different colors:

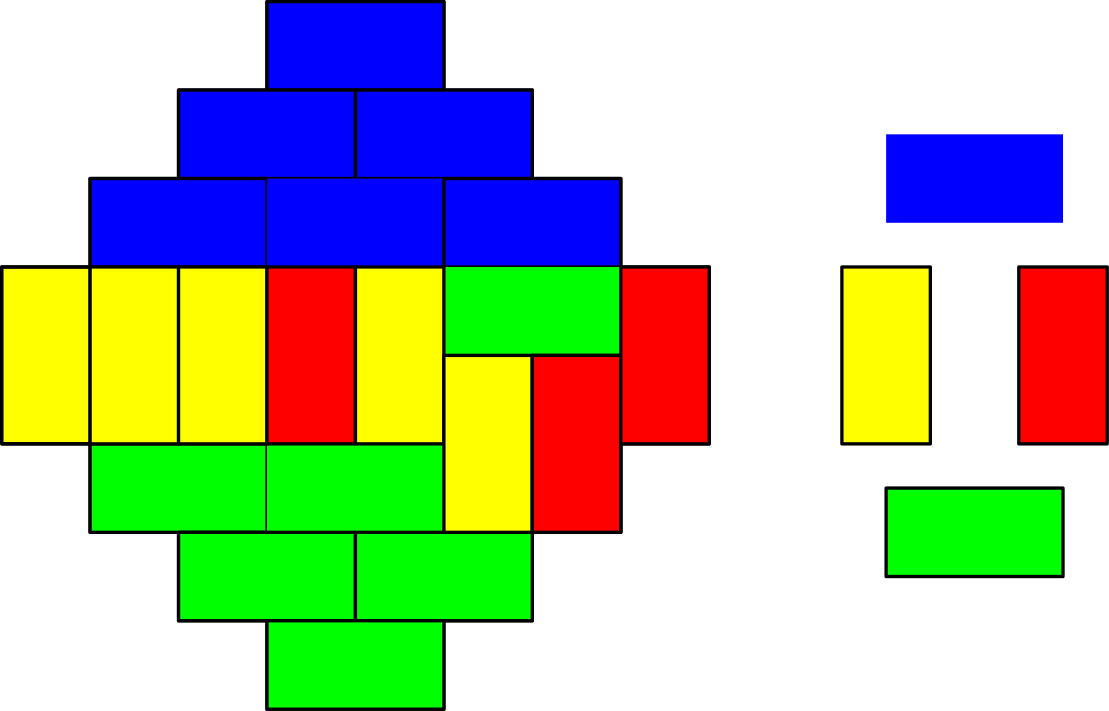
An even more visual way to parametrize domino tilings is known; it utilizes the notion of a height function that was introduced by Thurston [6]. It is an integer-valued function on the vertices of the underlying square lattice defined up to an overall additive constant. Along edges of the dominoes it changes by plus-minus one every step of the lattice according to the following rule. It increases by one if the square to the left of the traversed edge is black (in the checkerboard coloring), and decreases by one otherwise:

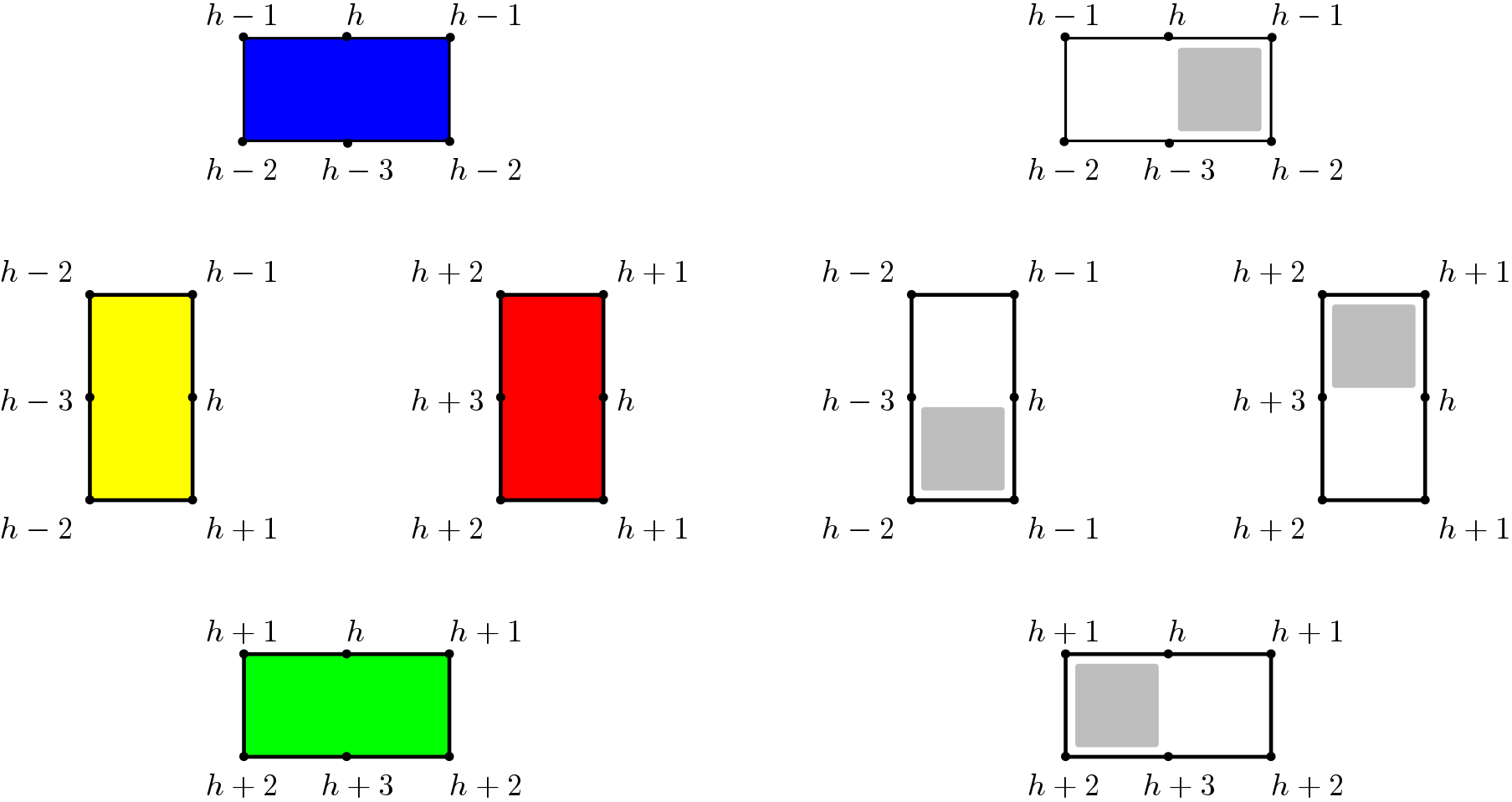
Thinking of the values of the height function as coordinates in the third dimension, the dominoes looks as follows:

Whenever a simply-connected (i.e., without holes) planar domain is tilable, the height function that obeys this rule can be defined on all vertices of the domain. The corresponding 3d image of the domino tiling from above then looks like this:

Random domino tilings of Aztec diamonds of large sizes demonstrate a number of interesting features, see [4] and references therein. Here is a 3d representation of a perfect sample drawn from the uniform distribution:

A perfect sample for a different distribution known as the "two-periodic Aztec diamond" can be seen below. Its distinctive feature is a "gaseous phase" that can be seen as a star-like almost flat region in the middle of the limit shape. This image was made possible thanks to a code written by Sunil Chhita for drawing perfect samples from two-periodic Aztec diamonds (via Propp's generalized domino-shuffling [5]), and we are very grateful to him for letting us use his code.

Higher periodicity of weights can lead to multiple gaseous regions, cf. [1], [2], and references therein. The following picture corresponds to periods 2 and 3, and it has two gaseous regions (the sample was generated by the same code as the one above; we thank Tomas Berggren for showing us how to use it for this purpose).

3d simulations and brief descriptions of other phenomena arising in random tilings of Aztec diamonds can be seen on this page, due to Dan Betea.

References.

1. T. Berggren and M. Duits, Correlation functions for determinantal processes defined by infinite block Toeplitz minors. Preprint, 2019. arXiv:1901.10877

2. P. Di Francesco and R. Soto-Garrido, Arctic curves of the octahedron equation, J. Phys. A: Math. Theor. 47 (2014) 285204. arXiv:1402.4493

3. N. Elkies, G. Kuperberg, M. Larsen, and J. Propp, Alternating-sign matrices and domino tilings (part I), Journal of Algebraic Combinatorics 1 (1992), 111-132. arXiv:math/9201305

4. K. Johansson, Edge fluctuations of limit shapes. Preprint, 2017. arXiv:1704.06035

6. W. Thurston, Conway's tiling groups. American Mathematical Monthly, Mathematical Association of America, 97 (1990), 757-773.